RANDOM FATIGUE
Characterisation of Dynamic Loading by PSD (Power Spectrum Density)
In many areas of technology random loads occur, but these can be described using statistical analysis because there are certain regularities.
For example, studies of aircraft, ships or road vehicles have shown that the excitation at certain frequencies has particular intensities and can be described by the power density spectrum. Using the finite element method, it is comparatively easy to determine the behaviour of structures under excitation using a power density spectrum.
This provides a comparatively easy to perform structural analysis, which has the decisive advantage over static analysis that the dynamic system properties are taken into account as a function of the excitation frequencies.
First, the simplest case of stochastic excitation in one direction only is described. Simultaneous excitations in several directions are similar in principle and will be discussed below.
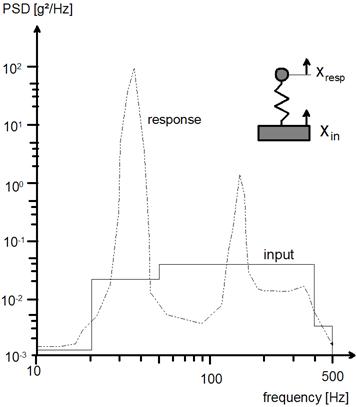
An example of a typical presentation is shown in the figure above. It shows the power density (power spectrum density) of a system excitation xin (e.g. excitation of a vibrating table, input) and a system response of the acceleration (movement of a point of the structure, response) in g²/Hz as a function of frequency. In reality, the structure to be tested is mounted on a test table instead of a point mass.
Unlike a point mass, real structures have a large number of points with different movements, for each of which a power density spectrum of the acceleration can be measured and calculated. The occurring stresses can also be shown for each point as PSD and a fatigue life calculation can be carried out accordingly.
The practical procedure for fatigue life calculation is shown in the following flow chart. It is assumed that the component to be analysed is placed on a vibrating table, which is assumed to be a rigid body.

Experimental Method
An experimental method uses, for example, a vibration table to which the structure to be tested is attached. A power density spectrum is specified as the excitation for the vibration table, and the component attached to it is accelerated accordingly at its contact points with the vibration table.
Such power density spectra can be found in standards for shipbuilding, aircraft construction or electronic components, and are used to define important test procedures that the component must withstand without damage.
Of course, it is also possible to test the component to failure and obtain an experimental result for the fatigue life. Usually, however, the component is not driven to failure, but only the successful demonstration of fatigue strength by surviving the test without damage. In order to obtain an estimate of safety, this test is often used to calculate the " fatigue life consumption ". This is done by measuring the strains at the points at risk and using this data to calculate the fatigue life. The measured strain curves as a function of time or the power density spectrum of the measured strain (stress) form the basis for a fatigue life calculation.
Computational Approach
The experimental approach can be completely replaced by computational methods. For this purpose, an FE model of the structure is created and the excitation by the acceleration is simulated by calculation. The acceleration is also specified by the power density spectrum of the acceleration in g2/Hz, similar to the experimental approach.
The natural frequencies and mode shapes are calculated for the system. These are important intermediate results that allow a plausibility check. It is essential that all masses and stiffnesses are entered correctly.
As a result, the RMS values of the stresses for a given frequency range are obtained for each node of the FE structure and from this the power density spectrum of the stresses can be determined in MPa2/Hz.
From the power density spectrum of the stresses, a frequency distribution of the stress amplitudes can now be calculated for each node, which is then used as the basis for a fatigue life calculation (see flowchart above).
Characteristic Parameters of a Dynamic System
A very important elementary parameter is the effective value or RMS value (Root Mean Square), which is defined as follows:

The stochastic parameters can be related to the frequency f in the unit Hz or to the angular frequency in the unit 1/s. The relationship between angular frequency and frequency is given by:

The square of the RMS value corresponds to the area under the power density curve. Other important parameters of the vibration behaviour are given by the spectral density moments (see figure).
To determine them, the area element Ø(f)df must be multiplied by the "lever arm" fn. For the zero order moment Hn, n=0, first order n=1, etc. up to n=4.
It is crucial that the following important parameters can be determined with these moments H0,H1,H2,H3,H4.
- RMS as above
- Number of zero crossings per second
- Number of peaks per second ( corresponds to the number of cycles per second)
- Irregularity factor I

It is possible to classify the dynamic behaviour of the system on the basis of the non-uniformity factor, distinguishing the following four cases, as shown in the figure.

Provided that the system behaviour is stochastic, stationary and ergodic, the appropriate method for calculating the amplitude spectrum can be selected depending on the non-uniformity factor.
SINE CURVE WITH CONSTANT AMPLITUDE AND FREQUENCY (I=1)
As this is not a stochastic process, the methods described below are not suitable for forming a collective. Instead, the behaviour is deterministic and a single-stage collective can be easily determined..
Narrow-Band Process (I~1)
The distribution is calculated using the following equation:
![]()
![]()
S is the amplitude, i.e. twice the amplitude. The index NB means Narrow-Band.
Broadband Process (0<I<1)
The distribution is calculated according to Dirlik [ ] using the following equation:

with:
Z = ![]() I =
I = ![]()
![]()
![]()
![]()
![]()
![]()
![]()
S is the amplitude, i.e. twice the amplitude.
Procedure of the Computational Analysis
Specification of the excitation for the vibration table:
Calculation of the eigenfrequencies and eigenmodes of the system (modal analysis) using FEM
Calculation of system response (PSD of stress for each node)
Determination of a damage-equivalent collective
Performing a fatigue life calculation.
An example with FEMAP shows the procedure in detail.